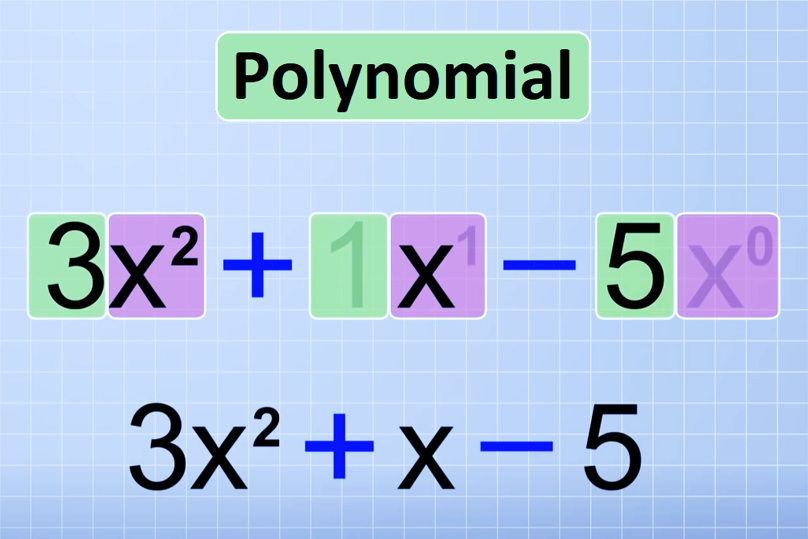
A type of expression which is used to represent or express various types of numbers in different fields of mathematics and calculus can be defined as a polynomial. An expression can be regarded as the representations of values on the L.H.S (left-hand side ) and R.H.S (right-hand side ) with the help of a sign, i.e. ‘=’. One unique fact about polynomials is, the exponents are whole numbers. Those numbers which are positive and do not contain any fraction or decimal can be defined as whole numbers.
For example, 1, 3, 5, 7, 4, etc. Therefore, all these numbers should be the exponents of the variables. Let us take an example: 3x.x + 2 is an expression where x is the variable, 3 is the coefficient, and 2 is regarded as the constant. In this article, we will try to cover some basic aspects of a polynomial such as types of polynomials, degree of a polynomial, some important terms related to it, and do a detailed analysis about it.
Degree of a Polynomial
We know that polynomials are regarded to be one of the most significant and vital parts of mathematics and calculus. Similarly, the degree of a polynomial is also very important as it finds the maximum number a function can have. The degree of a polynomial can be defined as the highest power of a variable in a polynomial equation/expression.
For example, 3x.x + 2 (polynomial equation) here, the variable (x) is considered to check the degree of a polynomial, the constants (2) and coefficient (3) are ignored. There are various types of polynomials based on degrees such as linear polynomial, cubic polynomial, quadratic polynomial, quartic polynomial, quintic polynomial, and many others. We shall discuss the types of polynomials based on degrees in the coming section.
Types of Polynomials
As mentioned above, a type of expression which is used to represent or express various types of numbers in different fields of mathematics and calculus can be defined as a polynomial. There are various types of polynomials such as monomial, binomial, trinomial, and so on. The following are the types of polynomials:
- A type of polynomial which is made up of a single term can be defined as a monomial. The term has been derived from the words of Greek where mono means ‘single’. For example, y, 5x.x, 3x ,etc.
- A type of polynomial which is made up of two terms can be defined as a binomial. We already know that ‘bio’ means two. For example, y – 8, 3x.x + 2, 4x + 3 etc.
- A type of polynomial which is made up of three terms can be defined as a trinomial. For example: y – x – z, 3x.x + 2y – 5 and so on.
Types of Polynomials based on Degrees
The degree of a polynomial can be defined as the highest power of a variable in a polynomial equation/expression. As mentioned above, the variables are to be considered for the polynomials based on degrees. Some of the types are as follows: linear polynomial, cubic polynomial, quadratic polynomial, quartic polynomial, quintic polynomial, and so on. The following are the types of polynomials based on the degrees:
- A polynomial with the degree ‘0’ can be defined as a Constant polynomial. For example, 3, 4, 7, 8, etc.
- A polynomial with the degree ‘1’ can be regarded as a linear polynomial. For example, y – 8, x + 3 etc.
- The polynomial with the degree ‘2’ can be defined as a quadratic polynomial. For example, 2x.x – 4x + 8.